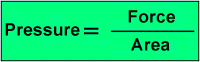 |
Stated using conventional abbreviations: (p for pressure, F for force and A for area) |
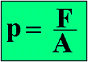 |
The official SI unit of calculation is the pascal, which is a pressure of one newton per square meter (Pa = N/m²). This very small unit is rarely used for practical calculations. Atmospheric pressures are calculated in hectopascals (hPa), while tire pressures, stacking pressures etc. are usually calculated in kilopascals (kPa) or megapascals (MPa).
In the pressure formula, force is above the fraction line, which means that, given an identical area, a larger force will result in a larger pressure. Area is beneath the fraction line, i.e. the smaller the area, the greater the pressure and vice versa.
If the maximum permitted stack pressure in containers is not known, it may relatively easily be determined. Point 3.1.7 of the CTU packing guidelines provides an indirect indication of how this is done:
-
Stowage planning should take account of the fact that CTUs are generally designed and handled assuming the cargo to be evenly distributed over the entire floor area. Where substantial deviations from uniform packing occur, special advice for preferred packing should be sought.
| Payload in kilograms | Force in newtons | Internal length in meters | Internal width in meters | Pressure in pascals | Pressure in kilopascals |
| 18,370 | 180,209.70 | 5935 | 2370 | 12,811.77 | 12.81 |
| 21,780 | 213,661.80 | 5895 | 2393 | 15,146.08 | 15.15 |
| 26,700 | 261,927.00 | 12,033 | 2352 | 9,254.84 | 9.25 |
| 29,650 | 290,866.50 | 12,069 | 2350 | 10,255.45 | 10.26 |
Example calculation of permitted stack pressure in containers
In the literature, values of 14 kN/m² for 20' containers and of 10 kN/m² for 40' containers are frequently stated as maximum floor loading values. However, as has been shown, this value varies from container to container and may easily be calculated. The maximum stack pressure in a container would be the same as the maximum container floor loading value if the container were packed absolutely evenly.
Official pressure units are only very rarely used for calculations in the transport sector. Since it is masses which are handled, pressure is often assumed to be the product of dividing mass by area:
Instead of the official units, units such as kg/ cm², kg/m², metric tons/m² or the English-speaking world's psi (pounds per square inch) are used. While, strictly speaking, this is incorrect, such units are widely used in practice and are more readily comprehensible to many of the personnel involved. The following relations apply when converting such units into the official units and vice versa.
- Hectopascals (hPa) correspond to the old unit millibar (mbar).
- One bar corresponds to 100 kPa or 0.1 MPa.
- Kilogram/square centimeter (kg/cm²) roughly corresponds to one bar or 100 kPa or 0.1 MPa.
- One kilogram/square centimeter corresponds to 10 metric tons/m²
- One kilopascal (kPa) = 1000 N/m² and corresponds to 100 kg/m² or 0.1 metric tons/m².
- One kilogram/square centimeter roughly corresponds to 14 lb/square inch (psi).
The stacking crush pressure is the pressure exerted by an item of cargo on underlying items of cargo or components.
| Pallet structure | Force in newtons |
Area of base in m² |
Crush pressure kN/m² = kPa |
 |
9,810 | 1000 | 9.81 |
 |
9,810 | 0.432 | 22.71 |
 |
9,810 | 0.288 | 34.06 |
 |
9,810 | 0.0864 | 113.54 |
 |
9,810 | 0.0576 | 170.31 |
Table: Crush pressures for different pallets
Crush pressures are significantly higher for some pressboard pallets and similar types. Unless appropriate measures are taken to distribute the pressure, it is not possible to guarantee that transport will proceed without damage.
 |
Elevated stacking crush pressures due to small contact areas |
Extremely high pressures may occur if buckets, pails, barrels and similar cargoes are packed without an intermediate layer. For example, if a barrel of a mass of 183.5 kg and a total area of the barrel rim of 90 cm² is placed on the floor, a pressure of 200 kN is generated. Assuming the barrel rim to have a width of 0.5 cm, offset stowage of another barrel on top will result in a contact area of 0.00015 m², generating a pressure of 12,000 kPa.
| Type of packing / contact areas | Force [N] |
Area [m²] |
Pressure [kPa] |
|
 |
 |
1,800 | 0.0090 | 200 |
 |
1,800 | 6 x 0.5 cm x 0.5 cm = 1.5 cm 0.00015 m² |
12,000 | |
The only way forwarding personnel can prevent damage due to static mechanical stresses is by taking steps to distribute the pressure, as it is not possible to modify the shipping packages themselves or to change the masses involved. However, many warehouses and packing centers do not hold stocks of appropriate materials in the form of walking boards, hardboard, wooden dunnage etc. Appropriate intermediate layers are often not used due to ignorance, for reasons of false economy or other motives.
It is essential to take the nature of the shipping packages into account. A skilled appraisal of the external appearance, such as the base of cases, cargo units and pallets, can provide valuable information as to how to load them. Efficient handling methods using lifting gear or ground conveyors usually require the use of beams or squared lumber beneath or between the items of cargo and such use can produce damaging levels of pressure.
Even strapping packages may cause damage due to excessive pressure if edge protectors are not used to distribute the pressure in order to offset the weakness of the packages.
Very high pressures are generated by narrow or point bearing areas. Negative effects are magnified by multiple tiers or the angles of rest which arise, for example, when stowing pipes, rolls or similar cargoes.
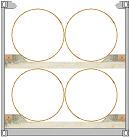 |
 |
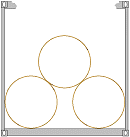 |
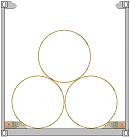
| Packing in tiers | Cantline 1 | Cantline 2 |
Packing in tiers generates extremely high pressures, as the pipes are only bearing on narrow strips corresponding to the edge lengths of the lumber. The resultant stack pressures are distinctly higher than in either of the two cantline stows. Due to the greater angles of rest or spread, cantline stow 2 generates greater forces than cantline stow 1. Further explanations in this respect may be found in the section, "Basic stowage methods".
The crush pressures bearing down on underlying layers of packages or the container floor is increased by the ship's motion. It is often forgotten that pressure does not only act downwards from above due to the force of gravity, but may also act laterally due to dynamic stresses. In this case, the packages are pressed against one another or the container walls. This pressure and in particular slipping and subsequent collision of inadequately secured packages may result in damage.
Very few packers anticipate "home-grown" static mechanical stresses due to inadequate cargo securing, but such stresses are predictable: if cargo stacks can move, critical and dangerously high pressures may arise as tipping occurs due to the resultant very small contact area.
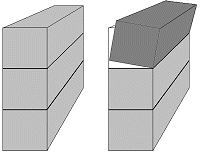 |
"Home-grown" static mechanical stresses |
The reduction in contact area with a simultaneous increase in pressure may cause the edges of the packages to cave in, resulting in damage to the shipping packages and collapse of the stack. In particularly unfavorable cases, this may result in the loss of whole batches or even of the container.
The actual tipping, collision or collapse of packages will be addressed in the dynamic mechanical shipping stresses section.